Eight-piece tablebases – a progress update and some results
Many readers will be familiar with tablebases, software that effectively plays perfect chess in certain endgame positions. If you’re new to the subject, though, refer to my introductory Adventures with endgame tablebases. As discussed in that blog series, the two most comprehensive tablebases currently are the Lomonosov and Syzygy, both of which cover all possible endgames with up to seven pieces. In the years since the releases of these databases, programmers have been building on the eight-piece version, which of course requires tremendous computer resources. One such developer, Marc Bourzutschky, has written a fascinating article-length update on the progress of his work, published on the Dutch endgame-study site, ARVES. Marc was the co-discoverer of the famous seven-piece position in which it takes White over 500 moves to force a win. Now he reveals which portions of the eight-piece tablebases have been completed and presents some of the most interesting positions found.
As tablebases advanced in solving more complex endgames, researchers were able to determine the longest possible forced win in positions with a total of three to seven units. It was observed that as the number of pieces increases in these record positions, the length of the perfect-play solution extends dramatically. This exponential growth pattern – the number of moves roughly doubles for each piece added – has been dubbed Haworth’s Law. It has been used to extrapolate that a thousand or so moves are needed to win in the record eight-piece position (and many thousands of moves for nine and ten pieces). However, Marc’s progressive results suggest that the Law may not hold up, even at the eight-unit stage. Here are some passages from his article on the issue:
One key discovery by YKMB [Yakov Konoval and Marc Bourzutschky] is the so far longest winning line of 517 moves in the 7-man endgame kqnkrbn, more than twice the length of the previous record of 243 moves in the 6-man endgame krnknn. An important question is at what point the chess board becomes so crowded that adding more pieces does not lead to longer winning lines due to the increased likelihood of shortening captures. My results suggest that we may already be at or close to this saturation point: The longest winning line for 8-man endgames without pawns appears to be “only” 400 moves.
…
After generating about 15% of the pawnless endings I’m quite confident to have captured the longest ones. While 15% seems like a small subset at first blush, most other piece configurations have large material differences between White and Black so that long lines are unlikely.
When Marc referenced his record seven-piece endgame with a winning line of 517 moves, that’s the position shown as the first diagram on this page of the Lomonosov site, even though the latter indicates it’s mate in 545 moves. The apparent discrepancy is due to the different tablebase metrics involved: Lomonosov employs the more intuitive depth-to-mate (number of moves to force mate), while Marc uses depth-to-conversion (number of moves to force a winning capture or mate). This sounds a little technical, but knowing their differences is helpful to appreciate the article more – see this section of the Wikipedia page on tablebases for a clear explanation of the two metric types. Thus in the new record eight-piece position, the 400-move sequence ends with a forced capture, not mate. As a rule, for any (non-drawn) endgame position, its DTM number is moderately higher than its DTC. So we expect the forced mate sequence of the record position to be above 400 moves, but not close to the maximum attained by seven units. Nevertheless, Marc surmises:
Even if 8-man pawnless endings don’t have any record lengths, that does not necessarily mean there may not be new record lengths for 9-man endings. Using the standard piece strength quantification (queen=9, rook=5, bishop=3, knight=3) the strength difference between White and Black for an 8-man ending is always even, while for a 9-man ending it is always odd. This may lead to new dynamics.
Here is the record position that is solved in exactly 400 moves. The round number is fortuitous, the two dark-squared bishops not so much! The solution, as is usual in such tablebase discoveries, defies human understanding and engine analysis.
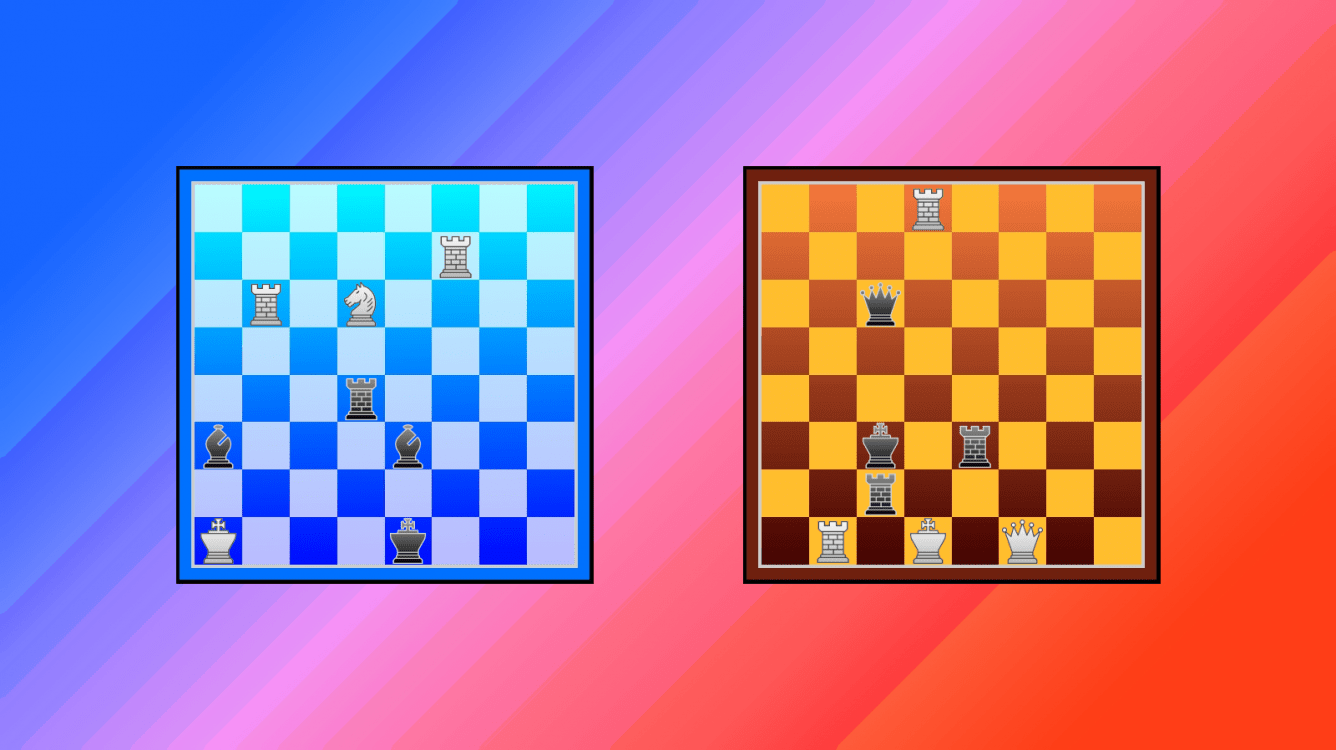
Marc indicates that his completed databases are too enormous to share, but he has made available a great number of files that summarise the results and showcase the special positions uncovered. Besides the length-record settings for various material configurations, he provides many mutual zugzwang positions (a topic I examined in Part 2 and Part 3 of my tablebase series). On the flip side, we see some examples of eight-piece endgame studies that are proven to be unsound by tablebase analysis.
One highlight among the mutual zugzwang arrangements is shown above. It’s a full-point MZ, a particularly curious type of situation where having the turn would be a fatal disadvantage for either side. The material is nicely balanced and happens to be the strongest possible without promoted units. Moreover, the brisk play is human comprehensible, and even that weakling known as Stockfish can solve it!
Source: 8-man Tablebase – First explorations


