Shortest games to stalemate and ‘Mr. Robot’ chess scene
In a type of chess problem known as synthetic games, the task is to construct a game starting from the normal array to reach a position that meets a certain objective. Since you control both sides, the play is no longer adversary, but the challenge becomes how to achieve the goal in the fewest number of moves. The Fool’s mate can be viewed as the answer to a synthetic-game problem, one stipulating for the fastest mate. All sorts of objectives are possible, however, such as reaching a position with only the two kings left, attaining a pattern of six white pawns on the same file, or finishing a game with a knight-promotion mate. Here we look at the task of producing stalemate in the quickest possible way, along with a few other related goals. Some of these record shortest games are quite famous, and two even featured in the acclaimed TV show, ‘Mr. Robot’. This drama series happens to be one of my favourites, and I will also examine the chess scene in question below.
Shortest stalemate game
The great Sam Loyd was a pioneer in many areas of chess problems, including synthetic games. He proposed various key tasks, and in some cases improved on the solutions of his contemporaries. His best-known composed game is the following one that effects stalemate in just 10 moves – a record that has held for 150 years and is surely unbreakable. 1.e3 a5 2.Qh5 Ra6 3.Qxa5 h5 4.Qxc7 Rah6 5.h4 f6 6.Qxd7+ Kf7 7.Qxb7 Qd3 8.Qxb8 Qh7 9.Qxc8 Kg6 10.Qe6. The move order is not precise since 4.Qxc7 and 5.h4 can be swapped, but such inexact play is normal in this genre. (By contrast, the move sequence must be unique in proof game problems – a modern offshoot of synthetic games – where the aim is to reach a specific position.)
Shortest stalemate game with no captures
What is the shortest stalemate game in which all 32 units remain on the board? The best answer is often attributed to Sam Loyd when proper credit goes to his American compatriot, Charles Wheeler. The latter was the first to devise this ingenious 12-move sequence: 1.a4 c5 2.d4 d6 3.Qd2 e5 4.Qf4 e4 5.h3 Be7 6.Qh2 Bh4 7.Ra3 Be6 8.Rg3 Bb3 9.Nd2 Qa5 10.d5 e3 11.c4 f5 12.f3 f4. Some alternative variations are possible, such as one that exchanges the places of Black’s pinning queen and dark-squared bishop. As T. R. Dawson explained in Ultimate Themes (1938), the task originated in 1882 and Wheeler published his 12-mover a few years later, surpassing earlier efforts. It wasn’t until 1895 that the task was re-set elsewhere, prompting Loyd and a few other problemists to rediscover Wheeler’s game and similar solutions.
Shortest double-stalemate game
Double-stalemate indicates a position where one player is stalemated while the other side, if given the turn, also has no legal moves. This kind of situation – probably unknown in practical play – works as an intriguing target of a synthetic game. In relatively recent time, the Italian composer Enzo Minerva established the record of 18 moves to obtain a double-stalemate. 1.c4 d5 2.Qb3 Bh3 3.gxh3 f5 4.Qxb7 Kf7 5.Qxa7 Kg6 6.f3 c5 7.Qxe7 Rxa2 8.Kf2 Rxb2 9.Qxg7+ Kh5 10.Qxg8 Rxb1 11.Rxb1 Kh4 12.Qxh8 h5 13.Qh6 Bxh6 14.Rxb8 Be3+ 15.dxe3 Qxb8 16.Kg2 Qf4 17.exf4 d4 18.Be3 dxe3. The game is impressively short for a task that entails juggling every single piece on the board.
Shortest double-stalemate game with fewest captures
Wheeler’s game above shows the paradoxical idea of a player stalemated without any unit captured. Wouldn’t it be even more remarkable to arrange a double-stalemate where both sides still possess their forces in full? Alas, that is impossible to achieve in a legal position, but another U.S. problemist, Gustavus Reichhelm, came closest to the task. He constructed a symmetrical double-stalemate position that contains 30 pieces, missing only two knights. The synthetic game itself involves a curious asymmetric element. 1.h3 g5 2.g4 Bg7 3.Nc3 Bxc3 4.Bg2 Ba5 5.b4 Nf6 6.Bb2 a6 7.Bxf6 b5 8.Be5 Bb7 9.Bg3 Nc6 10.Nf3 Na7 11.Nh2 c6 12.f3 d5 13.e4 Kd7 14.Ke2 Qb8 15.Qg1 Rc8 16.Rf1 Bd8 17.Be1 Kc7 18.Kf2 h5 19.a4 h4 20.a5 f5 21.c4 f4 22.c5 d4 23.e5 d3 24.e6. Despite the colour-symmetry of the diagram, the fastest sequence requires White to play an extra move.
Chess scene in ‘Mr. Robot’
‘Mr Robot’ (2015-2019) is a popular TV series that stars Rami Malek as a computer hacker and Christian Slater as a mysterious character nicknamed “Mr. Robot”. The show is a brilliant psychological thriller that has received plenty of accolades in its four-season run. In episode 4 of the second season, there is an extended scene of the two main characters competing over a chessboard. They played three games, all ending in stalemate to represent how neither could assert control over the other. As it turns out, they were recreating some of the classic synthetic games we have just seen. That the moves involved seem “bizarre,” or unlike those from a conventional game, is quite appropriate for a fantastical scene and a show best described as mind-bending! Indeed, without giving away spoilers, it makes perfect sense that they were playing composed games in which one person controls both sides…
Game 1 [timestamp 0:22] is Sam Loyd’s 10-move stalemate.
Game 2 [0:50] starts with 1.c4 h5 2.Qa4 a5 3.h4 Ra6 4.Qxa5 Rah6 5.Qxc7 f6 6.Qxd7+ Kf7 7.Qxb7 Qd3. We don’t see the rest of the play, but it’s easy to infer how the game concludes: 8.Qxb8 Qh7 9.Qxc8 Kg6 10.Qe6. Evidently this is just a version of Loyd’s game, with a different opening. What a pity that the show’s creators didn’t utilise Wheeler’s 32-unit stalemate instead!
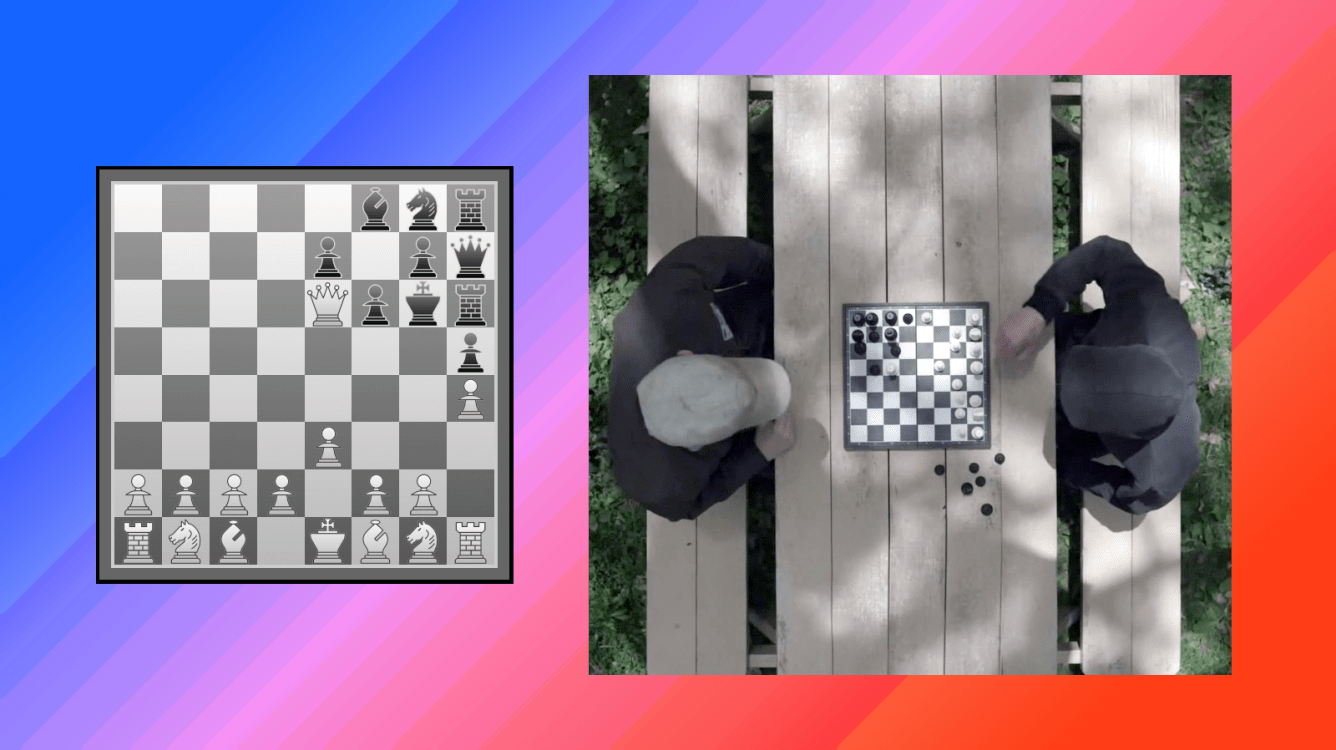
Game 3 [1:42] is Enzo Minerva’s 18-move double-stalemate. Enzo is an active problemist and he must be pleased to have his composition highlighted on a major TV series. Here is a screenshot that depicts the final position of the game.

An “extraordinary” moment in Gukesh-Ding WCC Game 13 and knight-switching proof games


